The Law of Cosines, which is sometimes also known simply as the Cosine Rule, can be very helpful in solving for unknown sides and angles in all types of triangles. A mathematician would write it out like this: c2 = a2 + b2 – 2abcos(C). Simple, right? No further explanation needed; let’s move on.
Just kidding! Although the average mathematician has had extensive practice putting the Law of Cosines to good use, the uninitiated may find that this string of letters and exponents is a bit much to process. Let’s break it down and see if we can make better sense of it.
Dedicated students may recall that the Pythagoras Theorem, which can be used to calculate values for right-angled triangles, reads a2 + b2 = c2. The Cosine Rule can be thought of as an extension of this theorem. Unlike Pythagoras’ discovery, though, this mathematical law holds true for all triangles, not just those that include right angles. It is most useful for finding one missing side when its corresponding opposite angle and the other two sides are known, or when all of the sides are known. It can also be used quite simply and effectively to find the angles of a triangle in which all three sides are known. Let’s take a look at a few examples to help make sense of why.
Example #1: Find an Unknown Side
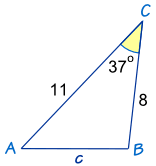
In this example, two sides and the angle that lies between them are known. The third side, “c,” is unknown. Thankfully, the Law of Cosines is set up perfectly to solve for the unknown variable; if instead we were solving for “a,” though, we would simply have to take the extra step of isolating the variable before proceeding. Since “c2” is already isolated on one side of the equation, though, all we have to do is plug in the known values. Once we’re done it should read: c2 = 82 + 112 – 2(8)(11)cos(37o).
Remember to follow the order of operations while completing these calculations. First, all of the exponents can be calculated independently of each other to get 64 and 121 for a2 and b2. The cosine of 37o can be approximated numerically as 0.798 for the purposes of solving the equation, leading to an equation that reads: c2 = 64 + 121 – 176 x 0.798, or c2 = 44.44. This is, of course, only an approximation.
If the situation calls for increased accuracy, estimate the number to further decimal places. Finally, taking the square root of both sides should leave us with c = 6.67.
The same process can be followed to find any angle in a triangle, provided all three sides are known.
When the formula is used to calculate missing angles it’s best to rearrange it to read:
cos(C) = a2 + b2 – c2 / 2ab and perform the calculations from there. Keep in mind that the Law of Cosines hasn’t changed in this circumstance; we’re just isolating the variable prior to plugging in all of the relevant information. The same strategy can be used to solve for a different angle, or a different side. Isolating the variable first does not change how the Cosine Rule is applied, it just makes it easier to perform all of the calculations correctly.