The word hexagon comes from the root words of hexa, or six, and gon which means plane shape with angles. Hence the strict definition of the shape. This means a hexagon is a six-sided plane shape with six angles.
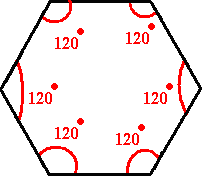
The properties of a gon are distinct in that each side is flat. The interior angles need to meet specific criteria to be considered a true hexagon. Each interior angle must be one hundred twenty degrees. This means that the sum of interior angles will always come to seven hundred twenty degrees.
Because the intersecting lines come together in such a specific way, the exterior angle will always be sixty degrees. This rule is a product of the fact that exterior angles form a linear pair. The measurement of these angels is true of all hexagons. Similar rules apply to all polygons regarding the number of sides and measurement of angles.
Area of A Hexagon
Because all hexagons share the same interior and exterior angles, finding the area of a hexagon is easier than most geometric shapes. In any geometric shape, the radius is a measurement from the center to a vertex created by intersecting lines. The radius of a hexagon is equal to the length of one side. Because of this, a regular hexagon can be broken into six equilateral triangles.
Finding the diameter is just as easy as finding the radius. The measurement of the radius simply needs to be multiplied by two, since the radius is half of the diameter. For example, if one side of the hexagon is four inches, so is the radius. This means the diameter would be eight inches. The radius is also called the apothem. Finding the perimeter is also easy if the value of the apothem is known. Because each side is the same as the apothem, that measurement can be multiplied by six and the value of the perimeter is found.
Calculating the area of a hexagon is a different matter altogether. Symmetry lends itself to finding the radius and diameter, but the size of the hexagon makes finding the area much more complicated depending on which formula is used. Finding the value of the apothem, diameter, or perimeter will make the calculation easier.
An easy way to find the area of a hexagon is to use the formula of Area = 1/2 x perimeter x apothem. Plugging in the values of the apothem and perimeter should be simple since the perimeter is six times the value of the apothem. With an apothem of nine inches, the answer to this formula would be two hundred forty-three inches, or Area = .5 x 54 x 9.
There are other formulas to find the area of a hexagon, each requiring a different piece of information. Some formulas require only a single piece of information, while the once shown above requires two. More complex formulas reveal the surface are of the hexagon and other information that can be used to find different geometrical measurements. By far, the formula above is considered the fastest and easiest method.
If certain measurements are still variables it will be necessary to find their value using additional formulas. At least one variable must be given a value for any of the formulas to produce a viable answer to the calculation of the surface area of the hexagon.